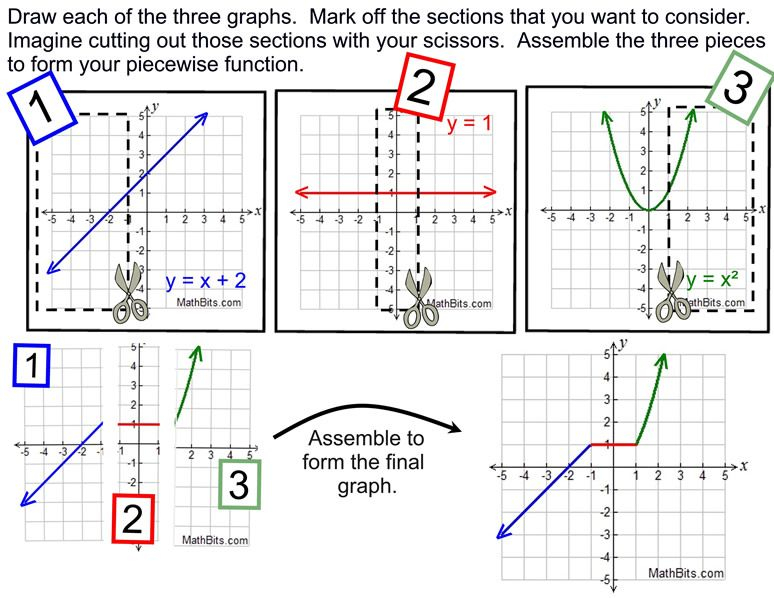
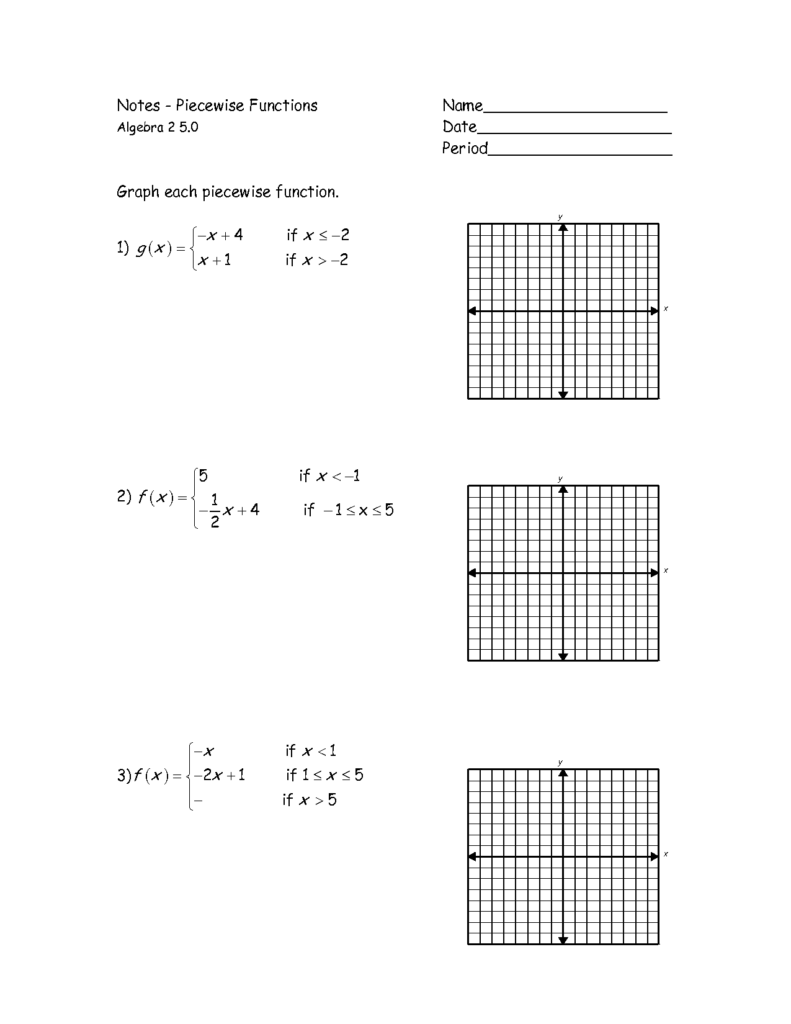
Graphing Piecewise And Step Functions Worksheet – If you’re looking for graphing functions worksheets, you’ve come to the right place. There are many types of graphing function to choose from. For example, Conaway Math has Valentine’s Day-themed graphing functions worksheets for you to use. This is a great way to help your child learn about these functions.
Graphing functions
Graphing functions worksheets are used to analyze data and draw graphs. Students will use graphing functions worksheets to compare data and solve problems. They will also learn about the different types of graphs. Some worksheets are focused on graphing inverse relations and functions. For example, one worksheet shows the graphs of a function, while another includes graphs of a function and the inverse of its domain.
The first step in graphing a function is to identify the x-intercept and y-intercept of the function. Then, students must complete the input-output table. They will then graph the function.
Identifying their shape
Identifying the shapes of different functions is one of the first steps in graphing them. In general, functions take positive values. If x=2, then the graph of function f(x), will take positive value. If x=1, then the graph graph of function k(x), will take negative value.
Graphs of different functions have similar shapes, but they can also have different shapes. If you have a graph of a function, you can identify the shape of the graph by its domain, range, and x-intercepts. You can then use this graph to calculate the values of the function.
Identifying their properties
Graphing functions have two basic properties: a domain and range. A real function has a domain and range of R. For example, y=3x is a real function. A one-to-one function is a function with one output value for each input value.
A continuous function has no jumps in its graph; instead, its values approach the value of x at every point. The opposite is true for functions with open intervals. An open interval is one that stretches from negative to positive. An open interval is a graphing function that has multiple domains.
An odd function has an inverse when x is replaced with a negative number. Its inverse is f(-x). An example of an odd function is a trigonometric sine function. It is also known as a cosecant function. It is possible to graph a linear function with a computer algebra system. This allows you to examine the properties of a function. You can then model the function by building a computational model of it.
Identifying their asymptotes
When graphing functions, you should identify their asymptotes. If the denominator is zero, the function has a horizontal asymptote. If the denominator is not zero, you should look for a vertical asymptote. Otherwise, you should avoid this type of asymptote. You can identify horizontal asymptotes by performing a highest order term analysis.
The point at which a function reaches its maximum value is called the asymptote. When this happens, the graph will be either horizontal or vertical. Horizontal asymptotes are marked with vertical dashed lines. If you graph a function that has a zero numerator, it can lead to asymptotes that are so close together that it is hard to tell the difference.
Graphing a rational function is similar to graphing a linear function. It will be necessary to compare the denominator’s degree with that of the numerator.
Identifying their vertex
Identifying their vertex is important for students to understand a graphing function. Students should be able determine the vertex of graphs by their x and y numbers. The vertex of a parabola is the point where the x and y values meet.
Students must identify the vertex when graphing quadratic functions. Then, they must convert the quadratic function’s standard form to its vertex form. They should also be able to locate the zeros in the quadratic functions. These graphing worksheets help students understand quadratic functions.